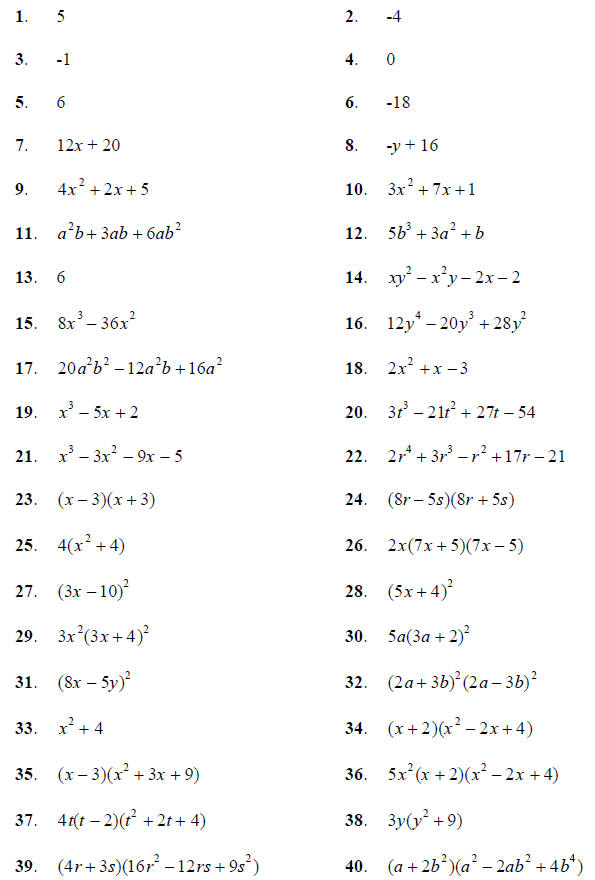POLYNOMIAL EXPRESSIONS

Definition

Example 1
Simplify the polynomial expression 7x² + 3xy − 4x² + xy − y² by combining like terms.

Solution

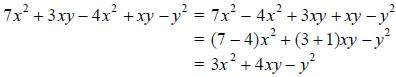
Example 2
Given x = -2 and y = 3, what is the value of xy² − 2xy .

Solution

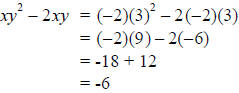
Procedure

Example 3
Find the product of (x² − y)(2x + y² − 3) .

Solution

Formulas

Description	Formula
Difference of two squares Perfect Square Trinomials Sum of two cubes Difference of two cubes

Example 4
Factor 9x² − 64y².

Solution
Since the expression 9x² − 64y² is the difference of two squares we have
9x² − 64y²
= (3x)² − (8y)²
= (3x −8y)(3x + 8y)

Example 5
Factor 16m² − 16mn² + 4n⁴ .

Solution
16m² − 16mn² + 4n⁴
= 4[4m² − 4mn² + n⁴]
= 4[(2m)² − 2 •(2m)(n² ) + (n² )²]
= 4(2m − n² )²

§2-2 PROBLEM SET

Evaluate each polynomial for the given values .
1. 3x ² + 2 where x = 1
2. − x² where x = -2
3. 2x² +3x − 3 where x = -2
4. 4t² − 6t + 2 where t = 1/2
5. x² y − 2x +1 where x = -1 and y = 3
6. 3ab − 2b² + 2 where a = 2 and b = -2

Simplify by combining like terms.
7. (7x − 2) + (5x + 22)
8. (3y + 4) − (4y −12)
9. (3x² − 2x + 7) + (x² + 4x − 2)
10. (5x² + 3x − 1) − (2x ² − 4x − 2)
11. (2a²b + 5ab² ) + (ab² + 3ab − a²b)
12. (5b³ − ab + 4a² ) − (a² − b − ab)
13. (3x² − 2x + 5) + (−3x² + 2x + 1)
14. (xy² + y − 2) − (x² y + 2x + y)

Multiply and then simplify .
15. 4x²(2x − 9)
16. 4y² (3y² − 5y + 7)
17. 4a² (5b² − 3b + 4)
18. (2x + 3)(x − 1)
19. (x − 2)(x² + 2x − 1)
20. 3(t − 6)(t ² − t + 3)
21. (x − 5)(x + 1)²
22. (2r² − r + 7)(r² + 2r − 3)

Factor completely .
23. x² − 9
24. 64r² − 25s²
25. 4x² +16
26. 98x³ − 50x
27. 9x² − 60x +100
28. 25x² + 40x +16
29. 27x⁴ + 72x³ + 48x²
30. 45a³ + 60a² + 20a
31. 64x² − 80xy + 25y²
32. 16a⁴ − 72a²b² + 81b⁴
33. x² + 4
34. x³ + 8
35. x³ − 27
36. 5x⁵ + 40x²
37. 4t⁴ − 32t
38. 3y³ + 27y
39. 64r³ + 27s³
40. a³ +8b⁶

§2-2 PROBLEM SOLUTIONS