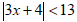March 17, 2009, Physics 126
• Functions and Graphs (1.2)
• Linear Functions , Slope, & Applications (1.3)
• Equations of Lines and Modeling (1.4)
• More on Functions (1.5)
• Algebra of Functions (1.6)
• Symmetry and Transformations (1.7)
• Linear Equations, Functions, & Models (2.1)
• Complex Numbers (2.2)
• Quadratic Equations , Functions, & Models (2.3)
• Graphs of Quadratic Functions (2.4)
• More Equation Solving (2.5)
• Solving Linear Equations (2.6)
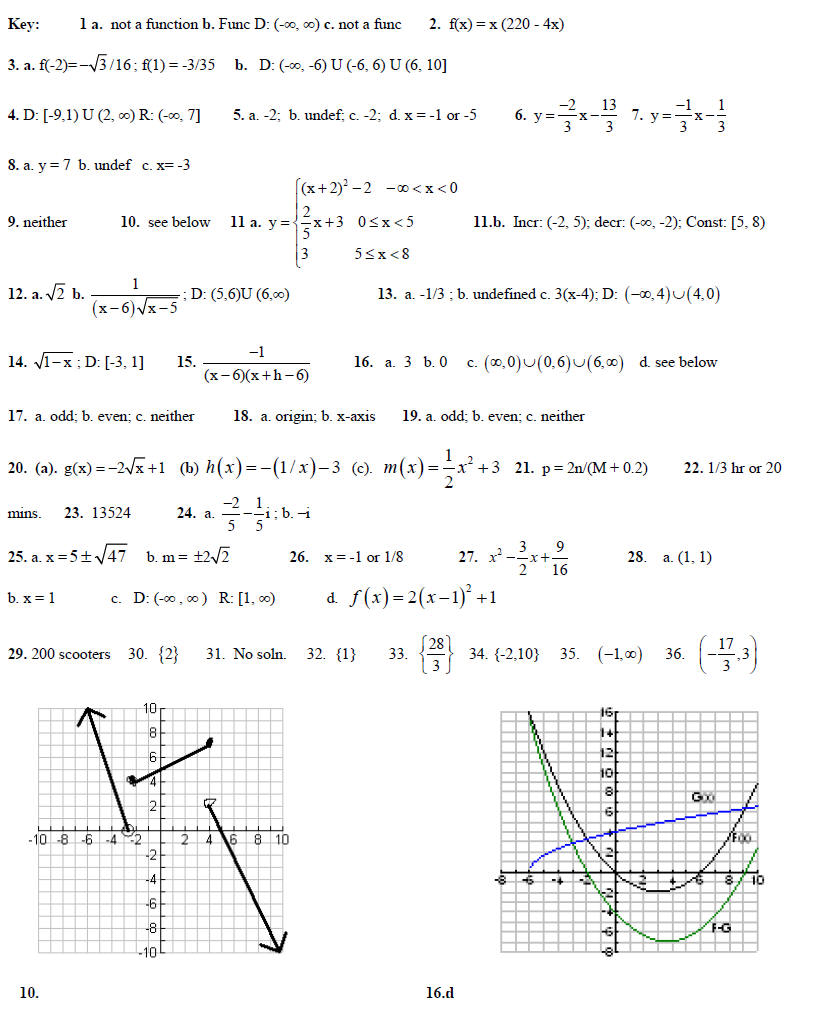
1. Determine whether each relation represents a function.
If so, identify the domain.
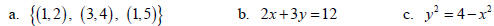
2. Find a function of x to represent the area enclosed in
the pen illustrated
at right, if 220 yards of fencing is used to construct the pen against the barn.

3. For  ,
,
a. find f (-2) and f (1)
b. Determine the domain of the function f(x).
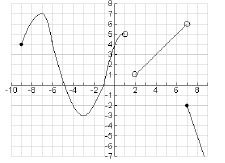
4. Determine the domain and the range of the function g(x)
whose graph
is given to the right. Write the domain and range in interval notation,
using the union symbol , U , whenever necessary.
5. From the graph in #4, determine the following:
a) g(-4) b) g(2) c) g(7)
d) Find all the x values such that g(x) = 1.
6. Find the equation of the line passing through (-5, -1)
and (-2, -3).
Express in y = mx + b form.
7. Find the equation of the line through the point (2, -1)
and perpendicular
to 9x – 3y = 4.
8.
a. Find the equation of the horizontal line through the point (2, 7).
b. Find the slope of the vertical line through the point (3, 4).
c. Find the equation of the line passing through the points (-3, 2) and (-3, 5)
9. Determine whether the lines are parallel,
perpendicular, or neither: 2x – 6y = 9 and 3x – y = 4.
10. Graph the piece-wise defined function
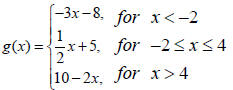
11.
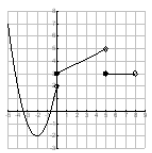
a. Write an equation for the piecewise function in the graph.
b. Indicate the intervals for which the function is increasing, decreasing, or
constant.
12. Let 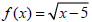 and
and
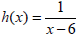
a. Find ( f / h)(7) .
b. Find (h / f )(x) and its domain.
13. Let 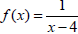 and
and
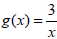 . Find
. Find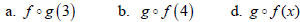 and
its domain.
and
its domain.
14. Let 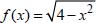 and
and
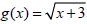 Find
Find 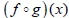 and
its domain
and
its domain
15. Compute and simplify the difference quotient
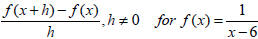
16. F(x) and G(x) are represented in the graph to the
right. Find:
a. (F + G) (3)
b. (Fο G) (10)
c. domain of (G / F) (x).
d. graph (F-G)(x)
17. Determine algebraically whether the following
functions are even, odd,
or neither even nor odd.
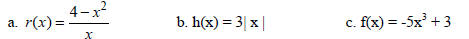
18. Which of the following are symmetric with respect to
the x-axis, the y-axis, or the origin ?

19. Classify the following graphs as even, odd, or
neither.
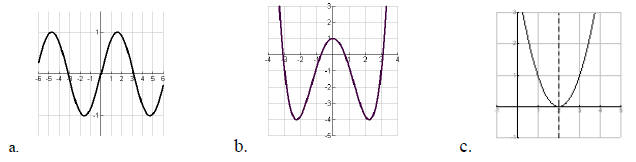
20. (a) The function g(x) has the same graph as that of
the function 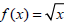 , stretched vertically by a
scaling factor of
, stretched vertically by a
scaling factor of
2, followed by a reflection about the x-axis, then shifted up by 1 unit.
Determine the equation of the function g(x).
(b) The function h(x) has the same graph as that of the
function 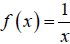 , reflected about the y-axis and
then shifted
, reflected about the y-axis and
then shifted
down 3 units. Determine the equation of the function h(x)
(c) The Function m(x) has the same graph as that of the
function f (x) = x^2 , stretched vertically by a factor of 1/2
, then shifted right 3 units. . Determine the equation of the function m(x).
21. Solve Mp = 2n – 0.2p, for p.
22. Cam and Pete are running a 10-km race. Cam runs at 12
kph and Pete runs at 10 kph.
In how many minutes will they be 2/3 km apart?
23. The population in Howard was 14,200 in 2000. This was
a 5% increase from 1995. What was the population in 1995?
24. Calculate
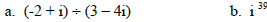
25. Solve:

26. Find the zeros for the function :
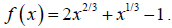
27. Add the appropriate constant to complete the square :
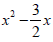
28. For the quadratic function, f(x) = 2x^2 – 4x + 3 find:
a) the vertex ;
b) the equation of the line of symmetry;
c) the domain and range;
d) Write the equation in the form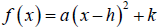
29. The cost C in dollars of manufacturing x scooters at
Scooter Productions is given by C(x) = 2x^2 – 800x + 92,000. Find
the number of scooters that must be manufactured to minimize the cost.
30. Solve:
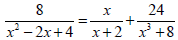
31. Solve:
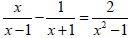
32. Solve:
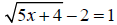
33. Solve:
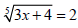
34. Solve:
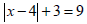
35. Solve and write interval notation for the solution
set :
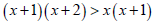
36. Solve and write interval notation for the solution
set: