Section 5.1: Integer Exponents

Recall the meaning of the exponential form of a number. 2³means “three factors of 2”. This can
be written in expanded form as 2•2•2 , and its value is 8.

In the exponential form, aⁿ, a is the base and  ⁿ is the exponent.

A. The Product Rule for Exponents states that “if you multiply two numbers (in exponential
form) that have the same base, you get a number (in exponential form) with the same base
and the exponent is found by adding the exponents of the two original numbers (in
exponential form)”. In symbols, we have:

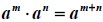
Ex. Multiply using the product rule: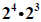

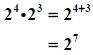
Ex. Multiply using the product rule: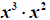

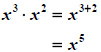
Ex. Simplify: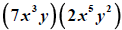

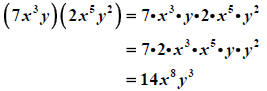
B. The Quotient Rule for Exponents states that “if you divide two nonzero numbers (in
exponential form) with the same base, you get a number with the same base and the
exponent is found by subtracting the exponent of the denominator from the exponent of the
numerator”. In symbols, we have:

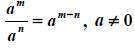
Ex. Simplify and write you answer using positive exponents: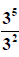

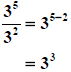
Ex. Simplify and write you answer using positive exponents :

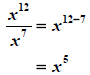
C. The Zero Exponent Rule states that “any nonzero number raised to the zero power is equal
to 1”. In symbols, we have:

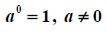
Ex. Simplify: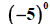

Since the base of this expression is −5 (which is not equal to zero), the zero
exponent rule applies.

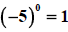
Ex. Simplify: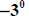

The base of this expression is 3 (which is not zero), so the zero exponent rule
applies, but only to the base of 3!

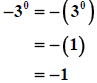
Ex. Simplify: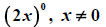

Here, the base is 2x and we know that x≠0, so  2x≠0 ,and ,so the zero
exponent rule applies to the base 2x.

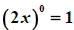
Ex. Simplify: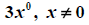

Here, the base is x and we know that x≠0, so the zero exponent rule applies
to the base x.

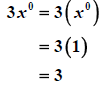
D. The Negative Exponent Rule states that “any nonzero base raised to a negative power is
equal to the 1 divided by the same base raised to the opposite of the negative power”. In
symbols, we have:

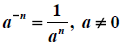
Ex. Simplify and write you answer using positive exponents: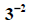

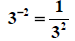
Ex. Simplify and write you answer using positive exponents: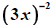

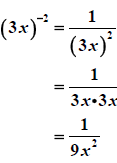
Ex. Simplify and write you answer using positive exponents: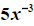

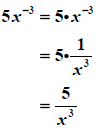
Ex. Simplify and write you answer using positive exponents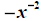

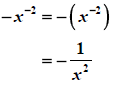
Ex. Simplify and write you answer using positive exponents: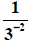

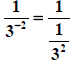
D.

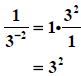
E. There are three Power Rules for exponents.

1. The Basic Power Rule for Exponents states that “any exponential expression that is
raised to a power is equal to an exponential expression with the same base and
exponent that is found by multiplying the exponents in the problem”. In symbols,
we have:

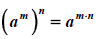
Ex. Simplify: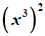

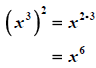
2. The Power of a Product Rule for Exponents states that “if you have a power of the
product of two (or more) numbers, raise each factor of the product to the new
power”. In symbols, we have:

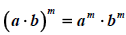
Ex. Simplify: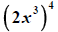
 

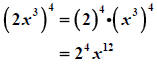
3. The Power of a Quotient Rule for Exponents states that “if you have a quotient of
of two nonzero numbers, raise the numerator and denominator each to the new
power”. In symbols, we have:

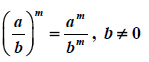
Ex. Simplify: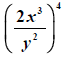

E.

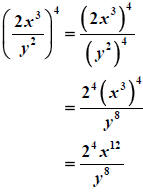
F. There are some Special Rules for exponents that are helpful in very specific situations. You
may use these if you like , but you are not required to know them. All problems can be
worked using the previously stated rules.

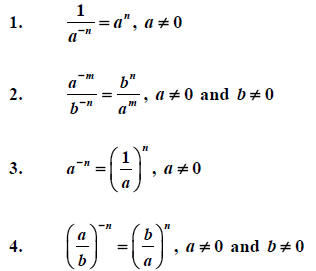
G. Assignment

Pages 294 – 297: 7 – 15 odd, 19 – 29 odd, 33 – 47 odd, 51 – 57 odd, 63 – 125 odd