Abstract
A visual method for adding fractions is introduced. The
similarities between this and another method are studied. A formal definition is
introduced in an intuitive and visual way. The multiplication is discussed as
well as analogies between addition and multiplication with union and
intersection of two sets. A final discussion on the philosophy of the method and
a comparison with another method for adding fractions is presented here.
1 Introduction
The teaching of addition of fractions at an elementary
school level has long a well known teaching challenge (Lappan-Bouck [4] and
Lamon [3]). It is widely recognized that there are at least two major
bottlenecks in the mathematics education of grades K-8: the teaching of
fractions and the introduction of algebra.” ([5, Page 1]) There are several
methods to approach the problem (Lamon [3]). Even though some have proved to be
successful, there is still the need of minimizing the difficulty of the
understanding of this addition process. This paper is intended to approach and
present the problem in a uniquely different way.
The method proposed here focuses on a visual method that
was discovered when trying to introduce fractions for the first time to a child
in the second grade.
In Sections 2 and 3, I discuss the meaning of a fraction,
its notation and the importance of understanding its value. In Section 4,
pictures allow us to visualize the addition of fractions which is done in
section 5. A comparison with other similar method of adding fractions is
discussed in section 6, and finally the multiplication of fractions is discussed
in section 7. The last sections are dedicated to the discussion on the
importance of giving a concise answer when teaching of adding fractions.
Analogies and the abstract meaning of the fractions are presented here.
2 What is one fourth?
What is one fourth? What is the actual value of one
fourth ? These are some of the typical questions a child might ask when he or she
is exposed to the concept of a fraction for the first time. The most formal
answers (which are believed to be the simplest) to these questions are presented
in this section. The child is expected to have a concept of multiplication
previous to any presentation of fractions.
There is an important number, it is called one, one unit,
one unit of a value and its symbol is 1. Let's think of number four for a
moment. Now there is a new number, called one fourth, that has the special
property four of this make one. The four of this make one property consists of
adding one number, the same number, the same number and the same number to
obtain 1. In our case, by definition, one fourth has the property that if we add
one fourth, one fourth, one fourth and one fourth together, we obtain 1. Another
way of saying this is four times one fourth is the same as one unit. The value
of this new number, one fourth, is still unknown ; but the number one fourth has
the above property that makes it different from others. It makes it unique. Its
symbol is 1/4 and the four of this make one property can be written as in figure
I.
Figure I
The four of this make one property in symbols and its simplified version
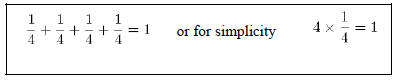
Among the numbers we knew so far (the integers), none of
them satisfies the four of this make one property. We can try with number 2 for
example, but it will impossible that 2+2+2+2 be equal to 1.
Let's now think of number 5, something similar happens.
There is a number called one fifth that satisfies a property which is not called
four of this make one anymore, no, the special property that one fifth satisfies
is called five of this make one. The symbol for one fifth is 1/5. The five of
this make one can be written as in
figure II.
Figure II The five of this make one property in symbols and its simplified
version
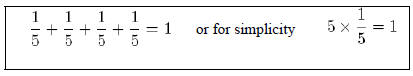
3 What is the true value of one fourth?
As we have met one fourth for the first time, we still do
not know its true value. We will never find the true value of one fourth among
the numbers we know. However, we can sense the value of one fourth as a small
portion of one unit. Let's imagine that we have one cake, our birthday cake
whose shape and size look like the figure 3.
Figure III
One Unit, one cake
Here our unit consists of a birthday cake of this size.
One fourth of our birthday cake will be a small portion that has the special
property four of this size make our birthday cake.
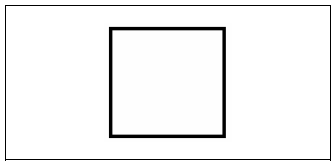
One fourth is not the small portion of the cake that looks
like figure IV, since four of these pieces make just an amount like in the one
shown in figure V, this amount has not the same size of our birthday cake.
Figure IV
A fake representation of one fourth
Figure V
Four fake pieces together
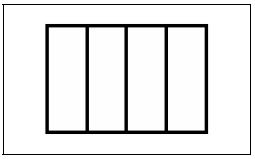
One fourth of our birthday cake looks like in figure VI.
Figure VI
A valid representation of one fourth
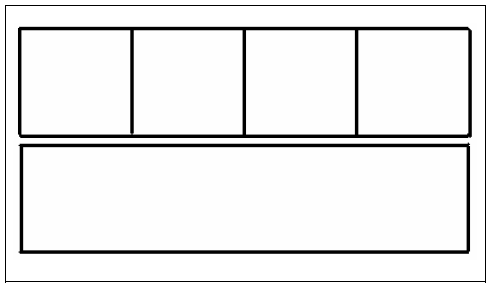
Indeed four of these pieces make an amount equal to the
one of our birthday cake, as it can appreciated in figure VII.
Figure VII
Four valid pieces together make our whole birthday cake
4 Representing fractions as shading areas
Let's now fix or establish a size, a measurement, and
clarify what our unit is; it will be a square, a particular square of any fixed
size. The size is not really important as long as we agree that from now on,
that will be the unit and it will not be changed. Three fifths will mean three
single one fifth-pieces. Three fifths looks like shading one fifth and another
one fifth and another one fifth together, like in the figure VIII.
Figure VIII
A colored representation of 3/5, the whole square represents our unit
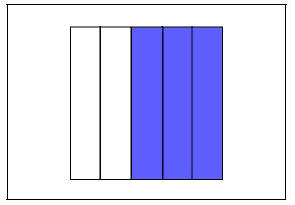
In figure VIII, just the shaded area is the one that
represents three fifths and the whole piece represents one unit (one unit of
cake for example). From now on, we will color transparent pieces of paper to
represent fractions.
5 Adding fractions
We add fractions of the type 1/12 and 3/12 in the
following way, we have a single one twelfth and three singles one twelfth,
therefore the sum of those will be four singles one twelfth, this is 4/12
(according to our definition of fractions). We will now study the addition of
fractions of different type. We now face the situation where we wish to add two
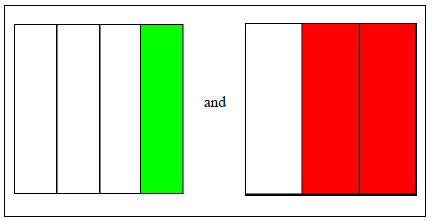
new numbers 1/4 and 2/3. Visually speaking, we want to add the quantities in
figure IX.
Figure IX
We wish to add the quantities shown
We now put them together by superimposing both squares and
rotate one of them ninety degrees. What we obtain is a grid of twelve little
rectangles. The big square represents one unit, one unit of cake. The cake has
been divided equally in twelve little parts. Some of them are red, other green
and the other ones have a mixture of red and green (yellow) as in the figure X.
Figure X
The colored area represents the sum of 1/4 and 2/3. The yellow being a
combination of both , counts double
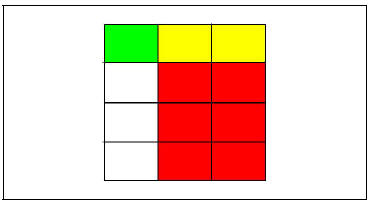
To find 1/4 + 2/3 we first count all the small rectangles
that are colored red, we then add the ones that are colored green, we finally
add the ones that have both colors, but these count double. We have then 1
(green) + 6 (red) + 2 (yellow) + 2 (yellow) = 11 colored pieces. Therefore the
answer to 1/4 + 2/3 is 11 out of 12, in other words, 11 /12.
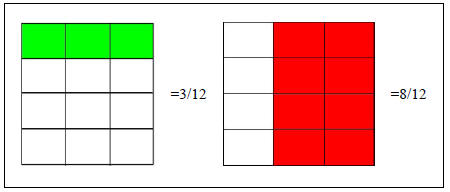
There is another way of approaching the same problem; in
fact this is the justification on why the previous method works. If we just look
at the superimposed squares and concentrate on the area that is or was green,
then this area can be estimated as three out of twelve little rectangles,
whereas the area that is or was red can be estimated as eight out of the twelve
rectangles, as in the figure XI. Therefore the sum 1/4 + 2/3 can be seen as,
3/12+8/12, which is, 11/12.
Figure XI
Grid showing equivalences of the desired fractions
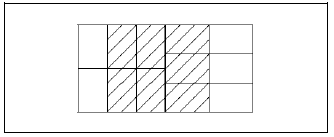
6 Comparing with Wu's method
H. Wu ([6, Page 55-56]) describes a geometrical
representation of 2/3 that is similar to the one presented here. When trying to
add 2/3 and 1/2, Wu combines the shaded areas in a horizontal way, and he
divides each piece into six equal parts creating a grid with each piece. By
aligning them horizontally, he uses these small rectangles as "yardsticks” to
measure the combined area and then he proceeds to count. In figure XII, the sum
of, the sum of 2/3 and 1/2 is illustrated via Wu's method ([6]) by aligning and
dividing each square.
Figure XII
Wu’s representation and addition of 2/3 and 1/2.
The method described by Wu is similar in the sense that a
grid is created. It is different in the sense that he has to align the pieces,
create the grid and do an analysis from there whereas in the method presented in
this paper, there is a superimposition and the counting is direct.
7 Multiplication of fractions
There is an easy way to compute the product of two
fractions via this method. It is understood that we define the product of two
fractions a/b and u/v as a times b over v times u. Suppose we wish to multiply
1/4 and 2/3. Visually speaking, we want to multiply the quantities in figure
XIII.
Figure XIII
We wish to multiply the quantities shown.
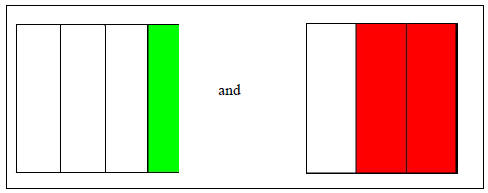
As before, we put them together by superimposing both
squares and rotate one of them ninety degrees. What we obtain is a grid as in
the figure XIV.
Figure XIV
The yellow area represents the product of 1/4 and 2/3
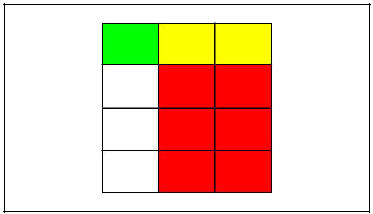
The result of the multiplication is the fraction
represented by the yellow area, that is, 2/12. The reason for this is that when
we superimpose the squares, we obtain a grid with as many rectangles as the
product of the denominators of our fractions , in our case, 12. Horizontally we
have as many colored strips as the numerator of one of our fraction and
vertically we have as many colored strips as the numerator of our other
fraction. In our case we have one green strip and two red strips. Hence the
amount of rectangles whose colors overlap can be counted as the product of our
numerators, in our case one green times two red produce 1x2 = 2 yellow
rectangles.
ETA Cuisenaire Company ([1]) has produced the set Picture
Grids (Fraction & Decimals ), Set of 60 ETA 020272.” This is a set of 60
translucent squared cards and each one of them has a fraction of it shaded with
a given translucent color. Each card represents a fraction just as the
representation described in this paper. The set of cards create the grid
described here by superimposing them. The package comes with a written
description on how to use the set, examples, pictures and problems. The written
description provides a full explanation on how to compute the product of two
fractions using the cards. However, the written description does not approach
the addition of fractions at all.
8 Analogy between union and addition, intersection and
multiplication
When we talk about the addition of two fractions, we
really mean putting together both fractions. This is precisely what the union of
two sets does, put together the sets. If the fractions are represented with
colored areas, then the union of those areas will represent the sum of those
fractions.
Analogously, the intersection of two sets provides the
proportion of one set inside the other one. The intersection of two sets, in
this case the colored areas, will represent a portion equal to the
multiplication of our fractions.
In fact, if we make an algebraic ring out of a family of
non-empty sets, the product of two sets will be given as the intersection and
the sum of two disjoint sets will be given as the union of these.
9 Philosophy of the method and the importance of an
abstract and formal answer
Wu ([6, Page 5]) defines a fraction as a division point on
the real line. This is merely a geometrical description, a graphical definition .
Lamon ([3]) writes: “As one moves from whole number into fraction, the variety
and complexity of the situation that give meaning to the symbols increases
dramatically. Understanding of rational numbers involves the coordination of
many different but interconnected ideas and interpretations. There are many
different meanings that end up looking alike when they are written in fraction
symbol...” I think that intending to explain the meaning of a fraction as a
result of combining old concepts such as the integers is a big mistake. People
look and look for an interpretation of fractions in real life via numbers that
they understand. A story that appeared at a newsletter for teachers at
University of Rhode Island ([2]) tells that on the first day of the teaching
career, an anonymous teacher defined a rational number to an eighth grade class
as a number that can be expressed as a ration of integers. A student asked the
teacher: What exactly are rations? How do rations differ from fractions? The
teacher was not satisfied with the answers given to the student and the teacher
consulted with some other teachers and texts. The result was confusion.
Wu ([6, Page 5]) mentions “...fractions tend to be
discussed at the source, in the sense that attention is invariably focused on
the interpretation of fractions in a real world setting. Since fractions are
used in many contexts in many ways, students are led through myriad
interpretations of a fraction from the beginning in order to get some idea of
what a fraction is. At the end, a fraction is never defined and so the
complexities tend to confuse rather than clarify...” Certainly the
interpretations and the contexts on which fractions are involved are endless,
however explaining a new concept in terms of the existing one is impossible. No
combination or algebraic combination of integers will ever produce 1.
When we only know the natural numbers or the integers, the
fractions are new numbers and we need to accept them as they are, new entities.
They are defined in terms of their properties and therefore these properties
make them unique. It is important to be able to recognize that the successful
answers are the ones that are short, realistic, and formal. The best job that a
teacher can do is trying to explain the formal definition in an intuitive and
logical way to the children. That is why when a child asks me, what is one
fourth? I answer; it is a new number that satisfies the property: four of this
makes one.
Finally, the goal of applying the visual method described
in this paper is not to gain expertise in all types of problems of addition of
fractions (such as adding three fractions, subtracting fractions , etc.) but to
gain intuition, clarity and formality about the concept of fractions and the sum
of two of them.
References
[1] E. Cuisenaire, Picture grids (fraction & decimals) set
of 60 eta 020272, 500 Greenview Ct. Vernon Hills, IL 60061, (2005).
[2] C. M. T. in Grades 712, Newsletter of the mathematics, Department of the
University of Rhode Island, 2 (1996), pp. 1{5.
[3] S. J. Lamon, Teaching Fractions and Ratios for Understanding. Mahwah, NJ:
Lawrence Erlbaum Associates, 1999.
[4] M. K. Lappan, Glenda & Bouck, Developing algorithms for adding and
subtracting fractions. The Teaching and Learning of Algorithms in School
Mathematics. Morow, L. J. & Kenny, M. J. (editors), Reston, VA: National Council
of Teachers of Mathematics., 1998.
[5] H. Wu, Some remarks on the teaching of fractions in elementary school,
Department of Mathematics # 3840, University of California, Berkeley Berkeley,
CA 94720-3840, (1999).
[6] , Chapter 2: Fractions., http://math.berkeley.edu/ wu/EMI2a.pdf, (2002). 13



