In this section we will review the techniques that we have
learned for solving different
types of equations. Before we can do that, we must go over the most important
step, and
that is factoring.
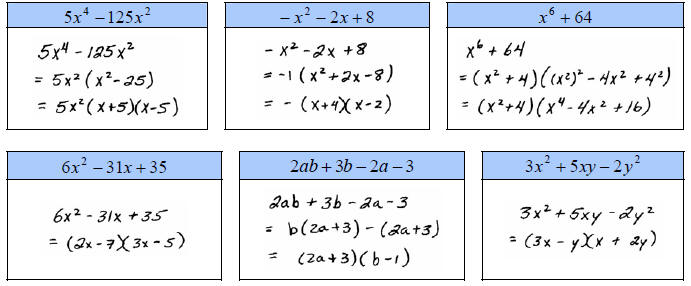
Guidelines for Factoring Polynomials:
1. Factor out the GCF (greatest common factor) first.
2. Four term polynomials – Factor by Grouping .
3. Trinomials – Factor by “guess and check.”
4. Binomials – Use the special products in this order:
* If a binomial is both difference of squares and
cubes we must factor it as
difference of squares first to obtain a more complete factorization.
* Not all polynomials factor over the real numbers, in this case,
Beginning
Algebra students can simply write “does not factor.” |
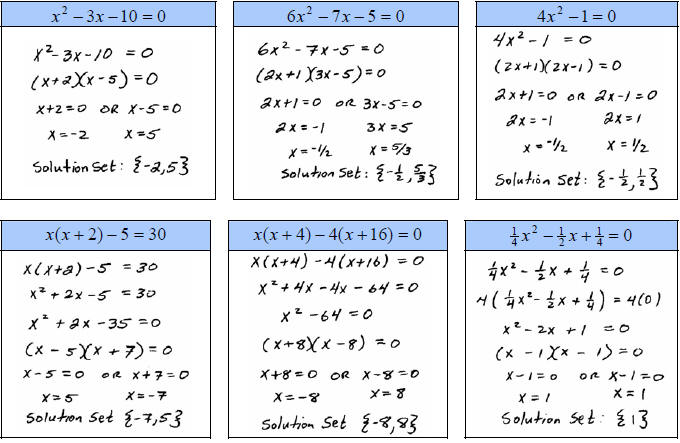
A. Factor
A common mistake is to set each factor equal to zero when
factoring , remember that we
only do that when solving.
Solving quadratic like equations requires us to
first factor then set each factor equal to
zero using that zero factor property.
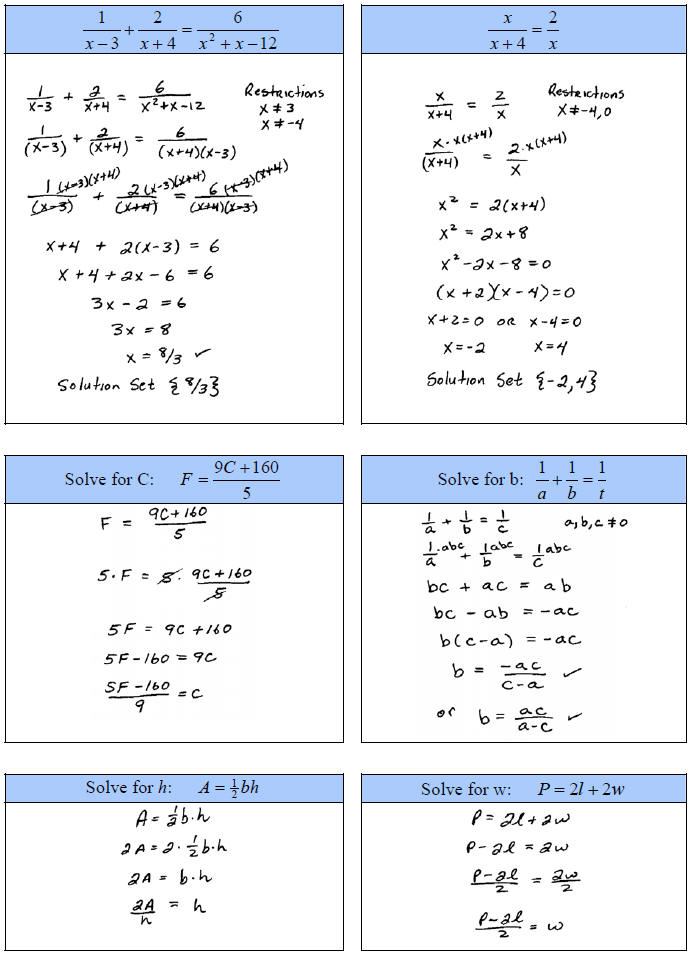
B. Solve
If we are given the solutions then we could work the
entire process in reverse.
Certainly we could check to see if the given solutions
solve the quadratic equations we
came up with.

If we are asked to solve an absolute value equation ,
lXl=n, we first isolate the absolute
value then set the argument X equal to ±n. We have seen this technique before
but the
difference here is that the resulting equations may be quadratic.
C. Solve

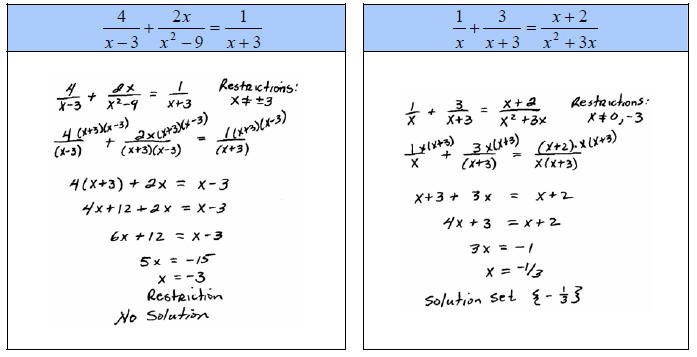
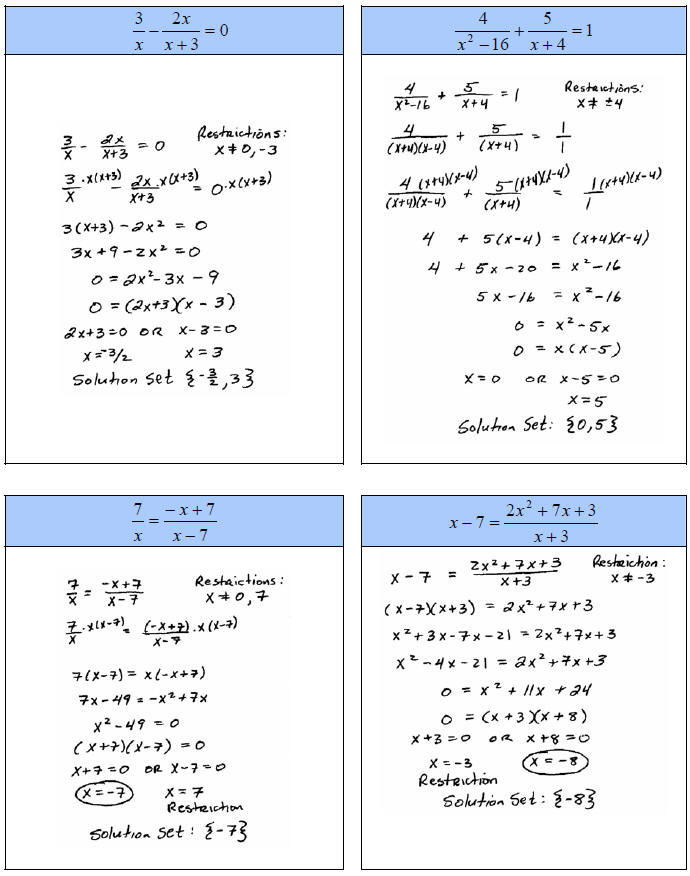
The technique for solving rational equations is the
same as well, multiply both sides by
the LCD and clear the fractions keeping a close eye on the restrictions.
Dividing by zero is undefined, with this in mind, whenever we see a variable in
the
denominator of an expression we need to consider that the variable could be a
number
that makes the denominator zero . A restriction on the variable is noted
so that the
variable in the denominator can not be assigned that value. If a solution to a
rational
equation turns out to be a restriction, simply throw it away. Do not include it
in the solution set.
D. Solve