Prerequisites: The prerequisite for MA101 is grade of C or better in
MA091, MA091A, or MA091D;
or appropriate score on the mathematics assessment test; or consent of
department. Assessment level:
RD 120
Course Description: Topics include development of Algebraic and
problem-solving skills and concepts
intended to prepare students for a mathematics foundation course. Topics include
linear, quadratic,
and exponential equations, functions and their applications, modeling and data
analysis.
Text and Supplies:
The optional textbook is Intermediate Algebra, 4th edition, Enhanced, by K.
Elayn Martin-Gay,
published by Pearson/Prentice-Hall, 2008. It is not the same as the MA091 text
or the MA101
classroom text used at Germantown. A notebook for taking notes and keeping
printouts is a great
investment. The homework assignments use the required software package MyMathLab,
The software access code can be purchased online or at one of the campus
bookstores.
Use of a graphing calculator will be required.
Homework Assignments:
Students must use the computerized software, MyMathLab, to complete their
homework assignments.
There are no due dates on these assignments, as you can work at your own pace –
but you must be
finished with the class on or before May 15, 2009, and that includes taking the
final exam. I will
provide target dates for you just so you can pace yourself.
Audit Policy:
A student who has registered for AUDIT in this class is just like the student
who registered for credit.
He or she MUST take the quizzes, attend classes and turn in completed homework
on time. The only
difference between an AUDIT student and a CREDIT student in this class is what
grade appears (or
does not appear) on the transcript. There is no other way for either one of us
to assess if you are
acquiring the knowledge.
Grading
Your grade will be based on your performance on homework assignments (worth up
to 70 in total),
five exams (worth up to 500 points in total), and a final exam (worth up to 130
points in total). You
may take retake any of the five regular exams for grades less than a 70%,
between 5/1/2009 and
5/15/2009. Exams are to be taken at the Germantown Assessment Center –
240-567-7739.
Your grade will be calculated in the two ways listed. The highest percentage
of the two will be used
as your overall course grade.
1) The average from the mandatory final alone.
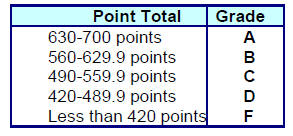
2) Your grade will be based on a maximum of 700 points. Grades will be
assigned as follows:
Withdrawal from Class
The last day to drop the class with a refund is February 1. The last day to
change to audit is February
15. The last day to drop the class without a grade is February 15. The last day
to drop the class with a
grade is April 19. I will NOT drop you for lack of effort…but you need to master
this material to move
on to MA110 or MA116.
Cancellation of Classes
If something forces the College to suspend classes or close, public service
announcements will be
provided to local radio and television stations as early as possible.
Support Services
There is a Math & Accounting Learning Center located in HT229 where tutoring
and support materials
are available. The hours for the fall 2008 semester were 8:30am – 9:00pm M – F
and 8:30am –
5:00pm on Saturdays. Hours for the spring 2009 have not been established as of
yet, but changes are
more likely to occur on Friday night and Saturday. The telephone number is
240-567-1947. Tutoring
and support are also available at Rockville and Takoma Park/Silver Spring
campus.
Policy on Academic Integrity: See the Student Code of Conduct – “Academic
Dishonesty and
Misconduct”. (Student Handbook)
The maintenance of the highest standards of intellectual honesty is the
concern of every student and
faculty member at Montgomery College. The College is committed to imposing
appropriate sanctions
for breaches of academic honesty. Academic dishonesty or misconduct can occur in
many ways. The
following are a few examples: plagiarism, cheating on examinations, copying a
friend's homework
assignment. This IS dishonest and a form of cheating, even if you worked on the
assignment together!
Working together IS okay; producing identical homework assignments is NOT
okay!
Disability Support Services
Any student needing an accommodation due to a disability should make an
appointment to see me
during my office hours. A letter from Disability Support Services (DSS)
authorizing your
accommodations will be needed. The DSS office is located in SA201 and may be
called on 240-567-
7783. DSS can also assist with making this publication is available in
alternative formats upon request
(i.e. Braille). A 24-hour TTY phone is available at 240/567-2133. The campus’s
main switchboard
also can receive TTY calls at 240/567-7000.
Course Objectives:
| TOPIC |
OBJECTIVE
Upon completion of this course, a student will be able: |
Linear Equations
& Functions |
Interpret the slope as a rate of change and in the context of
applications
Determine if a function is linear given the equation, a graph, a table
Interpret intercepts
Sketch the graph of lines
Find linear equations given the slope and a point; two points; a linear
model;
the equation of a related line |
| Introduction to Functions |
Determine if a relationship is a function, numerically, graphically,
or
algebraically
Apply and interpret algebraic, verbal, numeric, graphic definitions of
functions
Identify the domain of a function
Apply and interpret function notation including f(a); f(x)=b; the
intercepts |
Exponential Equations
& Functions |
Simplify and evaluate expressions containing rational exponents
Graph exponential functions of the form f(x) = abx
Solve exponential equations algebraically or by using a calculator
Solve exponential growth and decay problems |
| Logarithmic Functions |
Define logarithms and the relationship between logs and exponential
form
Define and apply the power rule to solve exponential equations
Apply properties of logs to simplify and evaluate logarithmic
expressions and
to solve logarithmic equations
Graph log functions of the form f(x) = logbx |
Quadratic Equations
& Functions |
Solve quadratic equations by factorization , the
square root method, the
quadratic formula
Analyze graphs of quadratic functions to include graphing parabolas ,
finding
the vertex, and finding and interpreting the intercepts |
| Applications |
Model real-world applications using linear,
exponential and quadratic
equations |
Modeling
& Data Analysis |
Create and analyze scatter plots from data |
| Graphing Calculator |
Enter a function and adjust the graphing window
Evaluate a function
Find the x- and y-intercepts
Find turning points on a graph
Find the intersection of 2 graphs |
| Systems of Equations |
Solve linear and nonlinear systems of equations |
Rational Functions
& Equations |
Identify the domain of rational functions
Simplify rational expressions
Solve rational equations |
| Linear Inequalities |
Graph linear inequalities in 2 variables
Graph compund inequalities in 1 variable |
| Applications |
To model real world applications using linear,
rational and quadratic
equations, and linear systems of equations in two variables |
Course Schedule:
Text
Chapter |
Target Date |
Topic(s) |
| 3 |
|
Hmwk 1 - Relations, Domain & Range |
| 3 |
|
Hmwk 2 - Functions |
| 2, 3 |
|
Hmwk 3 - Interval Notation |
| 3, 4 |
|
Hmwk 4 - Graphing Linear Functions |
| 3 |
|
Hmwk 5 - Slopes |
| 3 |
|
Hmwk 6 - Slope/Intercept Form |
| 3 |
|
Hmwk 7 - Graphing Using Intercepts |
| 3 |
|
Hmwk 8 - Review |
| 2 |
|
Hmwk 9 - Intro To Inequalities |
| 3 |
|
Hmwk 10 - Graphing Linear Inequalities |
| 2-4 |
2/7 |
Practice Test I (not for a grade)/Exam I |
| 6 |
|
Hmwk 11 - Solving Rational Equations |
| 6 |
|
Hmwk - 12 Rate/Time/Distance Problems |
| 6 |
|
Hmwk 13 - Work Problems |
| 7 |
|
Hmwk 14 - Introduction To Radicals |
| 7 |
|
Hmwk 15 - Simplifying Radicals |
| 7 |
|
Hmwk 16 - Radical Equations |
| 7 |
|
Hmwk 17 - The Pythagorean Thereom |
| 7 |
|
Hmwk 18 - Rational Exponents |
| 6, 7 |
2/28 |
Practice Test II (not for a grade)/Exam II |
| 5, 6 |
|
Hmwk 19 - The Zero Property |
| 8 |
|
Hmwk 20 - The Quadratic Formula |
| 8 |
|
Hmwk 21- Graphing Quadratic Equations |
| 8 |
|
Hmwk 22 - Using CALC Function & Quad.
Substitution |
| 5-6, 8 |
3/14 |
Practice Test III (not for a grade)/Exam III |
| 3, 9 |
|
Hmwk 23 - One To One Functions |
| 9 |
|
Hmwk 24 - Composition Of Functions |
| 9 |
|
Hmwk 25 - The Inverse Of A Function |
| 9 |
|
Hmwk 26 - Exponential Functions |
| 9 |
|
Hmwk 27 - Solving Exponential Equations |
| 9 |
|
Hmwk 28 - Intro To Logarithms |
| 9 |
|
Hmwk 29 - Properties Of Logarithms |
| 9 |
|
Hmwk 30 - Common Logs & The Change Of Base
Formula |
| 9 |
|
Hmwk 31 - Applications Of Logarithms |
| 3, 9 |
4/18 |
Practice Test IV (not for a grade)/Exam IV |
| 4 |
|
Hmwk 32 - Graphing Linear Systems of Equations |
| 4 |
|
Hmwk 33 - Solve Linear System by Substitution |
| 4 |
|
Hmwk 34 - Solve Linear System by Addition |
| 4 |
|
Hmwk 35 - Applications of Systems of Linear Eq'ns |
| 10 |
|
Hmwk 36 - Solving Non-Linear Systems |
| 4, 10 |
5/9 |
Practice Test V (not for a grade)/Exam V |
| ALL |
5/15 |
FINAL EXAM – Mandatory, Comprehensive |
The instructor may make amendments to this syllabus.



