Test 2A (10 Problems) Study sections of text and complete exercises
before attempting review
Chapter 2: Section 2.5 AND Chapter 3: Section 3.1 through 3.5 (omit 3.3)
1. [2.5] Finding Equations and Slopes of Horizontal and Vertical Lines
a. Find the equation of a horizontal line passing through (1, 2).
What is the slope of the line?
b. Find the equation of a vertical line passing through (0,-2).
What is the slope of the line?
2. [2.5] Analyze the Equation of a Line
Given 5x - 4y = 20 , find the slope and the y- intercept.
a. Rewrite in slope- intercept form :______________________________________
b. The slope of the line is________________________
c. The y-intercept is_________________
3. [2.5] Finding the Equation of a Line
Find the equation of the line passing through the given pair of points. Write
the equation in
slope- intercept form.
a. (-3,-2) and (5, 1) b. ( - 2,-2) and (4, 6)
4. [2.5] Finding the Equation of a Parallel or Perpendicular Line
a. Find the equation of the line containing (2, 1) and parallel to the graph of
the line shown
for problem 21 on page 188. [Page 171, in Second edition, blue cover, textbook.]
Simplify to slope - intercept form and circle the answer.
b. Find the equation of the line containing (3, -2) and perpendicular to the
graph of the line
shown for problem 22 on page 188. [Page 171, in Second edition, blue cover,
textbook.]
Simplify to slope- intercept form and circle the answer .
5. [2.5] Linear Models
a. The number of births (in thousands) in the United States in 1994 was 3797.
The number
of births (in thousands) in the United States in 1990 was 4158. Let B(x) be the
linear
function that expresses the number of births (in thousands) in the year x, where
x=0
represents 1990. (Source: National Center for Health Statistics).
i) Report the linear function.
ii) Use the function to predict the number of births in the United States for
the year 2000.
b. A flat tax has been proposed so that a person making $45,000 would pay
$3,100 in taxes,
while a person making $100,000 would pay $9,700 in taxes. Let T(x) be the linear
function that expresses the amount of tax paid on x dollars of income.
i) Report the linear function.
ii) Use the function to find what the tax will be for someone earning $80,000.
c. A rock is dropped from a 250 foot high building. After 1 second, the rock
is traveling at
39 feet per second. After 3 seconds, the rock is falling at a rate of 103 feet
per second.
Let R(x) be the rate at which the rock is falling after x seconds.
i) Report the linear function.
ii) At what speed will the rock be falling after 2 seconds?
6. [3.1] Solving Linear Equations Graphically
Solve the following equations using the intersection-of- graphs method .
Show what you entered for Y1 and Y2.
Round the solution to four decimal places.
a. 1.2x - 4.7 = 0.63 - 0.11x
b. 1.14x + 0.7 = 2.13 - 4.2 x
7. [3.2] Solving Linear Inequalities Algebraically

i) Solve the following inequalities.
ii) Graph the solution set on the number line.
iii) Write the answer using interval notation.
8. [3.2] Modeling with Linear Inequalities
For each problem, define the variable(s), translate
into a mathematical model and
solve. Interpret the results. Include correct units.
a. David has scores of 85%, 80%, 70%, 75%, and 75% on his
math exams. Use an
inequality to find the minimum score he can make on the final exam to pass the
course
with an average of 80% or higher, given that the final exam counts as three
exams.
b. Deanna earns a monthly gross salary of $800 plus a 15%
commission on her sales for
the month. Use an inequality to find the amount of the sales needed to receive a
total
income of at least $2000 per month.
c. Sears is having a sale on dress shirts. You buy the
first one at full price and then pay
half-price for the second, third, etc. articles of equal value. If the full
price of the shirt is
$12.98, up to how many can you buy for $51.92?
9. [3.4] Solving Absolute Value Equations
Solve algebraically .
a. |5 -3 x |= 6
b. |2x - 3| = 2
10. [3.4] Solving Absolute Value Equations Graphically
Use a graphical approach to solve the equations below.
Do NOT round solutions, if any.
a. a. 3.2- | x + 2 | = | x + 1| -1.4
b. - 2.7- | 2 x+ 1 | = | x - 2 |
Test 2B (11 Problems) Study sections of text and
complete exercises before attempting review
Chapter 3: Section 3.6 AND Chapter 4: Sections 4.1
1. [All] Vocabulary/Give an Example/True-False
This question will refer to the word list given in your outline and/or the
Highlight pages in
your textbook for this Test.
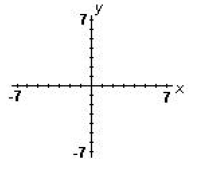
2. [3.6] Graphing Linear Inequalities
a. Graph the inequality: y ≤ 3 Plot
and label two points on the boundary
line with their ordered pair names. |
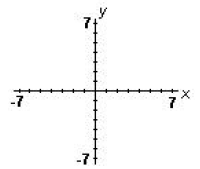
b. Graph the inequality: x > -2 Plot
and label two points on the boundary
line with their ordered pair names. |
 |
 |
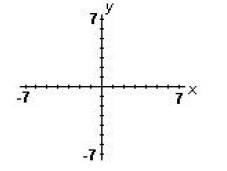
3. [3.6] Graphing the Intersection of Two Linear
Inequalities
a. Graph the inequality: 3x + 2y ≤ 6
Plot and label two points on the
boundary line with their ordered pair
names. |
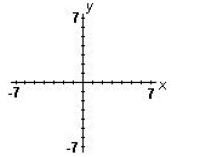
b. Graph the inequality: 5x - 3y < 15
Plot and label two points on the
boundary line with their ordered pair
names |
 |
 |
4. [4.1] Solving Systems of Linear Equations –
Substitution Method
Solve the following linear systems by the substitution method.

5. [4.1] Solving Systems of Linear Equations – Elimination
Method
Solve the following linear systems by the elimination method.

6. [4.1] Solving Systems of Linear Equation-Graphical
Method
Use the graphics calculator to solve the following linear systems.
Appropriate the solutions to two decimal places (nearest hundredth).

7. [4.3] Modeling With Systems of Linear Equations
For each problem, define the variable(s), translate
into a mathematical model and
solve. Interpret the results. Include correct units.
a. A movie theatre charges $5 for student tickets and $8
for adult tickets. If 175 tickets are
sold for a total of $1145, how many of each type of ticket were sold?
b. A coffee merchant wants to make a blend of coffee to
sell for $7 per pound. The blend
is made by mixing a coffee that sells for $4 per pound with one that sells for
$11 per
pound. If he wants to have 245 pounds of coffee how much of each type of coffee
should he use?
8. [4.3] Modeling With Systems of Linear Equations
For each problem, define the variable(s), translate
into a mathematical model and
solve. Interpret the results. Include correct units.
a. A printing company recently purchased $2000 worth of
new machinery so that they
could begin offering personalized T-shirts to their customers. The cost of
producing
each T-shirt is $5.00 and the company sells the T-shirts for $10 each. Find the
number
of T-shirts that the company must sell to break even.
b. The cost of a car rental at A1 is $25 per day plus
$0.25 per mile driven. The cost of car
rental at Z2 is $5 per day plus $0.50 per mile. Assume a rental of one day. How
many
miles must be driven for the cost to be the same for both companies?
9. [4.3] Modeling with Systems of Linear Equations



