The heart of these notes is the study of solutions to
cubic and quartic
equations, and this forms one of the most fascinating stories in the
history of mathematics. The discovery of their solutions in the sixteenth
century through the work of several Italian mathematicians was
the most dramatic advance to have taken place in algebra in centuries .
This section provides a mere sketch of the relevant history. For
more information, one can turn to the two volumes by B. L. van der
Waerden, Geometry and Algebra in Ancient Civilizations and A History
of Algebra From Al-Khwarizmi to Emmy Noether, and the references
contained within them. Also worth looking at is one primary source,
Gerolamo Cardano’s Ars Magna of 1545, as well as the book Algebra
in Ancient and Modern Times by V. S. Varadarajan, with its mix of
mathematics and history.
Every ancient civilization developed mathematics to at least some
degree, in part to serve the practical needs of measurement, construction,
and commerce. The work of the ancient Greeks may be the most
familiar, thanks to the codification of much of it by Euclid, but important
contributions were made as well within early Babylonian, Egyptian,
Hindi, and Chinese civilizations. Van derWaerden suggests, given
some of the common features of mathematics across these civilizations,
that a body of mathematical results existed within Central Europe in
the period around 3000 to 2500 BCE, and that this then spread to
Great Britain, the Near East, India, and China.
One particular problem that all these civilizations had addressed
was the solving of quadratic equations. Modern algebraic notation
was not available until many centuries later. As a result, mathematics
was often described through words and examples, sometimes relying
on geometry to express what we now consider to be algebraic concepts.
Through the working out of examples, a general method would be
implicitly laid out.
Let’s look at one example of the Babylonian treatment of quadratic
equations, as described by van der Waerden, using the translation by
the great historian of ancient mathematics and science, Otto Neugebauer,
of Old-Babylonian text BM 13901. A problem is stated as follows:
”I have subtracted (the side of) the square from the area , and it
is 14, 30.” What we are to find is the length of the side of the square.
Babylonians used base 60 in writing numbers, so 14, 30 is to be interpreted
as (14×60)+30, or 870. Thus, in effect, the problem is to solve
the equation
x2 − x = 870.
The general quadratic equation in this form would be
x2 − ax = b
and the quadratic formula would tell us in this case that one solution
is
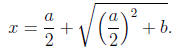
Substituting a = 1 and b = 870, we obtain x = 30.
The Babylonian text gives the same recipe in words. It says to take
1, the coefficient of the side of the square, divide it into two parts to
get 1/2, which is written as 0; 30 (recall that this is base 60 and think
of the semi-colon as a decimal point), form the product
0; 30 × 0; 30 = 0; 15
and add 14, 30. The result is 14, 30; 15, which has square root 29; 30.
The final step in the text is to add to 29; 30 the 0; 30 which was multiplied
by itself, obtaining 30 as the side of the square.
The point to be drawn from this, curious notation aside, is that
solving quadratic equations was well understood by Babylonians, and
other civilizations throughout the world, over 2000 years ago.
The mathematics that flowered in ancient Greece is surely the best
known of that developed in ancient civilizations, culminating with the
work of Diophantos of Alexandria on solving equations around 250
C.E. For the next thousand years, the principal developments in algebra
took place within Arab countries. Most notable of the Arabic
mathematicians is Muhammad ben Musa Al-Khwarizmi, who lived
roughly between 780 and 850 near Baghdad. As described by van der
Waerden, Al-Khwarizmi introduced the solution of problems by al-jabr
and al-muqabala. The process of jabr is that of adding equal terms
to both sides of an equation in order to eliminate negative terms. In
contrast, muqabala is the reduction of positive terms by subtracting
equal amounts from both sides of an equation. The combination of the
two words – al-jabr wal-muqabala– is sometimes used more generally to
mean performing algebraic operations, or simply the science of algebra.
Van der Waerden describes an example of Al-Khwarizmi’s. In modern
notation, one wishes to solve the equation
x2 = 40x − 4x2.
Using the operation of al-jabr, one adds 4x2 to both sides to obtain the
equation
5x2 = 40x
or
x2 = 8x.
From this, Al-Khwarizmi obtains the answer x = 8.
Al-Khwarizmi treats quadratic equations in his treatise The Compendious
Book on Calculation by al-jabr and al-muqabala.. I refer to
van der Waerden for more details. See also his treatment of the algebraic
work of two later Arabic scholars, Tabit ben Qurra and Omar
Khayyam (the famed poet).
The return of significant mathematical activity within Europe occurred
in Italy, no doubt because its cities were major trade centers
with connections to Arabic ports along the Mediterranean. In the thirteenth
century, Leonardo da Pisa, better known to us under the name
Fibonacci, wrote several mathematical works that were preserved. Of
special note for us is that he provided a treatment in the Liber Abbaci
of linear and quadratic equations, citing the work of Al-Khwarizmi. A
number of Italians continued to work on algebra in subsequent centuries,
but we may jump ahead to Fra Luca Pacioli, who lived from
1445 to 1514. His main work, Summa de arithmetica , geometrica, proportioni
e proportionalit`a, was published in Venice in 1494. Of note is
that in it he writes, with regard to cubic and quartic equations, that
”it has not been possible until now to form general rules .” This was
the setting as the sixteenth century dawned.



