1 Teaching objective(s)
The student will
convert decimals to fractions and fractions to decimals.
At completion of this
lesson, student will be able to convert decimals to fractions
and fractions to decimals with at least 70% accuracy.
2 Instructional Activities
1. The teacher will start the lesson by instructing each
student to suggest situations
for which they would want to express a fraction as a decimal, or to express a
decimal as a fraction . Have students discuss the responses. Examples may
include: as a decimal—for impact, as in advertising.
2. The teacher will open the lecture by explaining to the
students that 3 out of every
25 people are left-handed. The teacher will write the following fraction on the
overhead 3/25.
3. The teacher will ask each student if they are
right-handed or left-handed. After
doing so, the teacher will write the fraction on the overhead. The teacher will
explain the method for expressing a fraction as a decimal.
4. The teacher will explain that a fraction indicates
division, and instruct the student
to divide the numerator of the fraction by the denominator. The teacher will
model the method of using long division (pencil and paper) of 3/25 on the
overhead,
and the method of using the calculator, which is 3/25= 0.12. (Once the teacher
is
convinced students understand how to express a fraction as a decimal, encourage
them to work more efficiently by using calculators to find answers .)
5. The teacher will explain that in using both methods,
they were changing a
fraction into a decimal. The teacher will have students work with a partner
and change the following fractions into decimals using long division and the
calculator: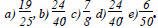 
The teacher will explain that
decimals to
fractions and fractions to decimals methods have to be illustrated on paper.
Students will discuss the answers among themselves. One student can take the
role of recorder and write the answers on paper, and the other student can take
the role of reporter and report to the class.
6. The teacher will then explain to students that
decimals, in turn, can be expressed
as a fraction. The teacher will explain that a decimal can be expressed as a
fraction with a denominator of the power of ten indicated by the place value of
the
final digit of the decimal , and then simplify the fraction. The teacher will
write
the following on the overhead—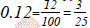
The teacher
will watch for students
who choose the incorrect power of 10 when writing a decimal as a fraction.
7. The teacher will write the following examples on the
overhead and discuss the
answers: a)0.45 and b) 0.8.
8. The teacher will have students work with a partner and
change the following
decimals into fractions: a)0.08, b) 0.78, c) 0.29, d) 0.225, e ) 0.10. The
teacher
will explain that all work must be shone. The students will discuss the answers
among themselves. One student can take the role of recorder and write the
answers on paper, and the other student can take the role of reporter and report
to
the class.
9. The teacher will explain to each student that they will
complete an activity
entitled-Skittles® Fun. The teacher will pass each student a package of
Skittles® . The teacher will instruct each student to open the package of
Skittles® and pour them on a sheet of paper. The teacher will tell students
not to eat the Skittles® . The teacher will first instruct each student to count
the total number of Skittles ® that were in the package. The student will write
this
number in their journal. The teacher will instruct each student to make groups
of
the different colors of Skittles®. The student will count the number of each
color
and express this number as a fraction of the bag. After expressing each group as
a fraction, the student will change the fraction into a decimal. The student
will
report their findings in their journal. The questions for the journal writing
will
be: 1. How many different colors are in a bag? 2. What is the ratio of colors ,
red
to blue, blue to green, green to yellow, and yellow to red?
3 Materials and Resources
Overhead projector
Pencil
Notebook
Textbook - Mathematics -Applications and Connections, Course (Glencoe, 1995)
Calculators –TI 73
Skittles® for each student
4 Assessment
Teacher observation of student participation
Peer evaluation
Student product -Skittles® Fun
Journal writing-recorded answers
