PERFECT NUMBERS: AN ELEMENTARY INTRODUCTION

JOHN VOIGHT

Abstract. This serves as an elementary introduction to the history and theory
surrounding even perfect numbers .

One would be hard put to find a set of whole numbers with a
more fascinating history and more elegant properties surrounded
by greater depths of mystery—and more totally useless—than the
perfect numbers.

—Martin Gardner [2]

The number 6 is unique in that 6 = 1+2+3, where 1, 2, and 3 are all of the proper
divisors of 6. The number 28 also shares this property, for 28 = 1 + 2 + 4 + 7 + 14.
These “perfect” numbers have seen a great deal of mathematical study —indeed,
many of the basic theorems of number theory stem from the investigation of the
Greeks into the problem of perfect and Pythagorean numbers [16]. Moreover, it
was while investigating these numbers that Fermat discovered the (little) theorem
that bears his name and which forms the basis of a substantial part of the theory of
numbers. Though it is rooted in ancient times , remarkably this subject remains very
much alive today, harboring perhaps the “oldest unfinished project of mathematics”
[17].

This paper surveys the history and elementary results concerning perfect numbers.

1. Early History

The almost mystical regard for perfect numbers is as old as the mathematics
concerning them. The Pythagoreans equated the perfect number 6 to marriage,
health, and beauty on account of the integrity and agreement of its parts.

Around 100 c.e., Nicomachus noted that perfect numbers strike a harmony
between the extremes of excess and deficiency (as when the sum of a number ’s
divisors is too large or small), and fall in the “suitable” order: 6, 28, 496, and
8128 are the only perfect numbers in the intervals between 1, 10, 100, 1000, 10000,
and they end alternately in 6 and 8. Near the end of the twelfth century, Rabbi
Josef b. Jehuda Ankin suggested that the careful study of perfect numbers was an
essential part of healing the soul. Erycius Puteanus in 1640 quotes work assigning
the perfect number 6 to Venus, formed from the triad (male, odd) and the dyad
(female, even). Hrotsvit, a Benedictine in the Abbey of Gandersheim of Saxony
and perhaps the earliest female German poet, listed the first four perfect numbers
in her play Sapientia as early as the tenth century:

JOHN VOIGHT

We should not leave unmentioned the principal numbers . . . those
which are called “perfect numbers.” These have parts which are neither
larger nor smaller than the number itself, such as the number
six, whose parts, three, two, and one, add up to exactly the same
sum as the number itself. For the same reason twenty-eight, four
hundred ninety-six, and eight thousand one hundred twenty-eight
are called perfect numbers [2].

Saint Augustine (among others, including the early Hebrews) considered 6 to
be a truly perfect number—God fashioned the Earth in precisely this many days
(rather than at once) to signify the perfection of His work. Indeed, as recorded by
Alcuin of York (who lived from 732 to 804 c.e.), the second origin was imperfect, as
it arose from the deficient number 8 > 1+2+4, this number counting the 8 souls in
Noah’s ark (Noah, his three sons, and their four wives, in Genesis, chapter 7) from
which sprung the entire human race [8]. Philo Judeus, in the first century c.e.,
called 6 the most productive of all numbers, being the smallest perfect number.

Throughout the centuries that followed, various mathematicians carefully studied
perfect numbers (see the continued extensive history given by Dickson [6] and
also by Picutti [14]). Up to the time of Descartes and Fermat, a sizeable pool of
important results—as well as much misinformation—had been collected.

2. Elementary Results

We now turn to some definitions and some elementary results.

Definition 1. The sum of divisors is the function 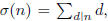, where d runs
over the positive divisors of n including 1 and n itself.

For example,

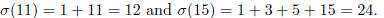
Definition 2. The number N is said to be perfect if 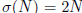. When
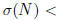
2N, we say N is deficient; when 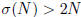, we say N is abundant.

The definition of perfect is equivalent to saying that the sum of the proper (or
aliquot) divisors of N is equal to N (we just do not add N itself to the sum). While
this may seem more natural, the central reason for using the function 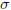is that it
possesses some very special properties. First:

Proposition 3. 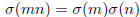whenever gcd(m, n) = 1.

Proof. If d is a divisor of mn, then by unique factorization we can represent d
uniquely as the product of a divisor of m and a divisor of n (since they have no
common factors). That is, every term of 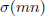(the sum of all divisors of mn)
occurs exactly once in the sum 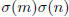(the product of all divisors of m and n).
The converse is also true: every such product is a divisor of mn, so the sums must
be the same.

This suffices to establish the proposition. It might be easier also just to appeal
to the very special way in which is defined as a divisor sum (for the details consult
[12]).

Hence 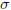is completely determined when its value is known for every prime-power
argument. (Such functions are, of course, the multiplicative functions.) This yields
the very useful:

Theorem 4. If 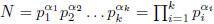is a factorization of N into primes,
then

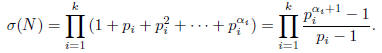
Proof. The only divisors of
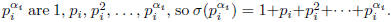
Recall the geometric series has the closed form

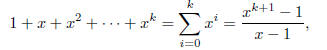
which implies that 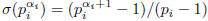. Since is multiplicative,

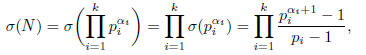
as claimed.

To give us just a little bit practice and feel for this formula for the sum of divisors,
we prove the following two short lemmas. If 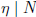it is clear tha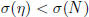t , but
an even stronger result holds:

Lemma 5. If 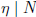, then

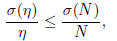
where equality holds only if η = N.

Proof. Note that if d | N then kd = N for some k, so k = (N/d) | N. This argument
works just as well in reverse, so we have that d | N if and only if (N/d) | N, which
implies that

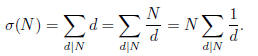
If η is a proper divisor of N, we have

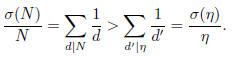
Otherwise, equality holds.

For free, we also have:

Corollary 6. If N is perfect,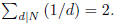

Proof. We just showed d | N is equivalent to (N/d) | N, hence

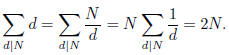
Cancelling N proves the corollary.

We assume henceforth that the reader is familiar with congruence arithmetic
and the fundamentals of group theory (consult [12] or [7] for some of the basics),
of which we will use often use various facts without proof.

3. Even Perfect Numbers

The first individual to really categorize the perfect numbers was the Greek mathematician
Euclid. He noticed that the first four perfect numbers are of a very
specific form:

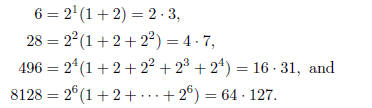
Notice, though, that the numbers 90 = 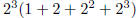= 8 · 15 and 2016 =
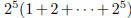= 32 · 63 are missing from this sequence. As Euclid pointed out,
this is because 15 = 3 · 5 and 63 = 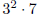are both composite, whereas the numbers
3, 7, 31, 127 are all prime.

As it appears in Book IX, proposition 36 of his Elements, Euclid writes: “If
as many numbers as we please beginning from an unit be set out continuously in
double proportion, until the sum of all becomes prime, and if the sum multiplied
into the last make some number, the product will be perfect.”

We state this observation in a slightly more compact form:

Theorem 7 (Euclid). If 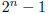is prime, then N = 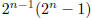is perfect.

Proof. Clearly the only prime divisors of N are 2ⁿ − 1 and 2. Since 2ⁿ − 1 occurs
as a single prime, we have simply that 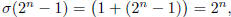and thus

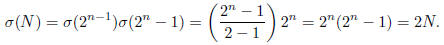
So N is perfect.

The task of finding perfect numbers, then, is intimately linked with finding
primes of the form 2ⁿ −1. Such numbers are referred to as Mersenne primes, after
the seventeenth century monk Marin Mersenne, a colleague of Descartes, Fermat,
and Pascal. He is credited with investigating these unique primes as early as 1644.
Mersenne knew that 2ⁿ − 1 is prime for n = 2, 3, 5, 11, 13, 17, and 19—and, more
brilliantly, conjectured the cases n = 31, 67, 127, 257. It took nearly two hundred
years to test these numbers.

There is one important criterion that hones in on the primality of Mersenne
numbers:

Proposition 8 (Cataldi-Fermat). If 2ⁿ − 1 is prime, then n itself is prime.

Proof. Note from before, xⁿ − 1 = (x − 1)(x^n-1 + · · · + x + 1). Suppose we can
write n = rs, where r, s > 1. Then

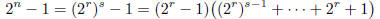
so that (2^r − 1) | (2ⁿ − 1) which is prime, a contradiction.

Note that the converse is not true—the number 2¹¹ − 1 = 2047 = 23 · 89, for
instance.

PERFECT NUMBERS

Must all perfect numbers be of Euclid’s type? Leonard Euler, in a posthumous
paper, proved that every even perfect number is of this type. Many ingenious proofs
of this fact exist.

Theorem 9 (Euler). If N is an even perfect number, then N can be written in the
form N = 2^n-1(2ⁿ − 1), where 2ⁿ − 1 is prime.

Proof. The first proof is Euler’s own [6]. Let N = 2^n-1m be perfect, where m is
odd; since 2 does not divide m, it is relatively prime to 2^n-1, and

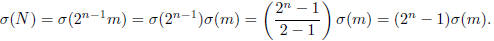
N is perfect so 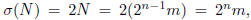and with the above, 2ⁿm =

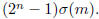
Let 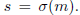We then have since 2ⁿ does not divide
2ⁿ − 1, it must divide s (because m is an integer), so that m = (2ⁿ − 1)q for some
q = s/2ⁿ. If q = 1, we have a number of Euclid’s type, for then m = 2ⁿ − 1 and
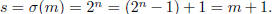Since 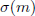is the sum of all of the divisors
of m, m = 2ⁿ − 1 must be a prime, and

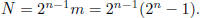
If q > 1, we retotal the sum of the divisors of m = (2ⁿ − 1)q. The factors of m
then include 1, q, 2ⁿ − 1, and m itself, so that

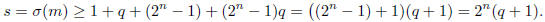
But this implies

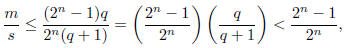
an impossibility, for we have previously established equality:
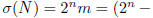
1)s implies that m/s =

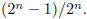
Proof 2. A second, simpler proof is given by Dickson [5]. From
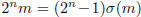
we note

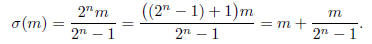
Since both 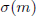and m are integers, so too must d = m/(2ⁿ − 1) be an integer.
Thus (2ⁿ − 1) | m and consequently d itself divides m.

But 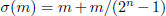= m+d is the sum of all of the divisors of m. How
can this be? Certainly 1 divides m, so are forced to conclude that d = 1; if this were
not the case, then we would have 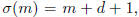a contradiction. Therefore,
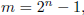and in partiular, m has no other positive divisors other than one and
itself, so 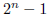is prime.

Proof 3. A third proof is given by McDaniel [10]. Since 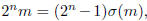every
prime divisor of 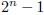must also divide m (for it is odd and cannot divide 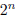). So
suppose 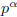divides 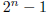with p prime.

By Lemma 5,

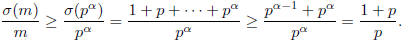
Hence,

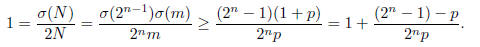
JOHN VOIGHT

This is only satisfied when the fraction on the right is zero , so that p = 2ⁿ − 1,
α = 1 and m = p, hence N is of Euclid’s type.

Proof 4. A similar proof is provided by Cohen [4]. Again we start with 2ⁿm =
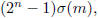where 2ⁿ − 1 | m. We then write

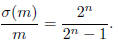
From the previous lemma,

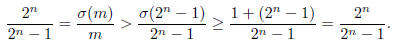
To sustain equality throughout this expression, we must have 2ⁿ − 1 = m and
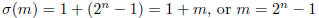is prime.

Proof 5. A fifth proof is given by Carmichael [3]. Let

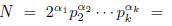
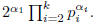Then

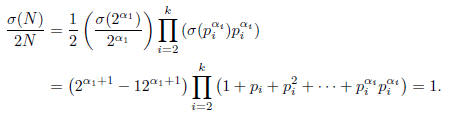
Write 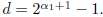Rearranging and dropping terms on the right, we have

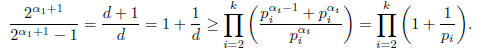
Note that 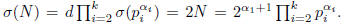Immediately, then,
some p_i must divide d, for the right-hand side has been completely factored into
primes and d is odd. If p_i is an aliquot divisor, then p_i < d and 1+1/p_i will exceed
the left side of the inequality . Hence p_i = d. But with that, if k > 2, the inequality
is again exceeded, so k = 2. Likewise, 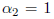and we have a number of Euclid’s
type.

Proof 6. As if that weren’t enough, a sixth and final proof is given by Knopfmacher
[9]. Very familiar now, we have

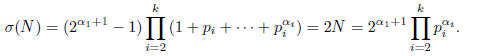
Since 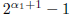cannot divide 2, it divides N, and we may write

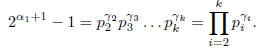
It follows that

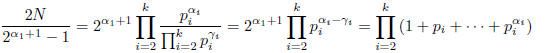
PERFECT NUMBERS

and therefore

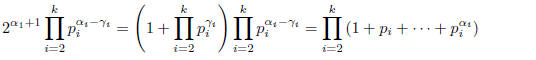
so

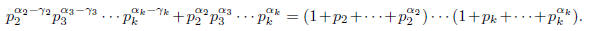
Since 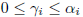for all i, expanding the product on the right will yield each of
the terms on the left. But there are the only two, which means that 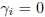for all
values of i except one; so then k = 2, and 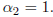The above equation then yields
simply

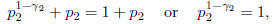
so 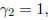and N is of Euclid’s type as claimed.

Even perfect numbers have a number of nice little properties. We say that a
number is triangular if it can be arranged in triangular fashion, that is, one atop
two atop three (and so on) arranged in a triangular lattice. While perfect numbers
can be written in a geometric expression `a la Euclid, they may also be derived from
arithemetic progressions:

6 = 1 + 2 + 3, 28 = 1 + 2 + 3 + 4 + 5 + 6 + 7, 496 = 1 + 2 + 3 + · · · + 31.

We state this result as follows:
Proposition 10. If N is an even perfect number, then N is triangular.

Proof. We have m triangular if 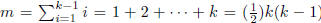for
some k. But note that

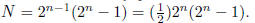
We can also write any perfect number as the sum of cubes:

Proposition 11 ([1]). If 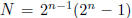is perfect then

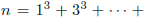
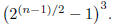
Proof. Recall the formula

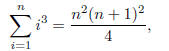
a fact which can be proved by induction. Let 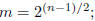we have then that

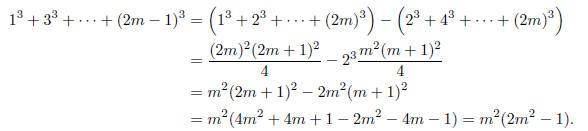
By substituting for m we get the desired result.

Perfect numbers have very unique representations. As first noted by
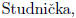
we have 6 = 110₂, 28 = 11100₂, 496 = 111110000₂, and 8128 = 1111111000000₂
written in binary; in general:

Proposition 12. If 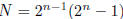is perfect and N is written in base 2, then
it has 2n − 1 digits, the first n of which are unity and the last n − 1 are zero.

JOHN VOIGHT

Proof. This follows immediately from how numbers are written in binary, by recalling
that

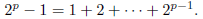
We also have the historically interesting fact:

Proposition 13. Every even perfect number ends in either 6 or 8.

Proof. Every prime greater than 2 is of the form 4m + 1 or 4m + 3. In the first
case,

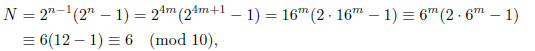
since by induction it is clear that 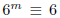(mod 10) for all m. Similarly, in the
second case,

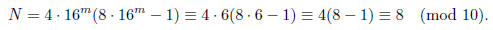
Finally, if n = 2, N = 6, and so we have captured all the possibilities.

Even perfect numbers exhibit another amazing property, first proven in 1844:
Proposition 14 (Wantzel). The iterative sum of the digits of an even perfect
number (other than 6) is one.

Proof. Let 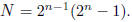The first claim we establish is that 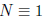(mod 9).
Now 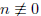(mod 3), for then 3 | n so n is not prime (N ≠ 6) and N is not perfect.
Hence 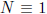or 2 modulo 3. Suppose we have the first case, and n = 3k + 1 for
some k. Now n is odd, so k is even and we could have written n = 6k + 1. Hence

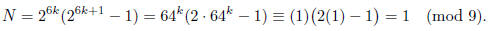
In the second case, n = 3k + 2 for some k, so as n is not even, k is odd and
n = 6k + 5. Thus,

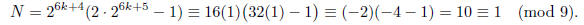
The work is now done, for the iterative sum of the digits of a number is merely
its congruence class mod 9. For if 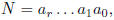where the a_i are digits, then the
sum of digits is

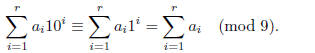
By iterating this sum we get the desired result.

Historically, there are a number of problems that arise in the investigation of
even perfect numbers. For instance, Fermat declared that if n is a prime, then
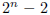is divisble by 2n. Of course, this immediately follows from the fact that
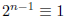modulo n if n is prime by Fermat’s little theorem.

Fermat also showed that if n is prime, then 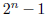is divisible by no prime other
than those of the form 2kn + 1. If q | 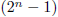for some prime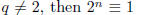
modulo q. Since we have a power of 2 equal to 1, the identity, the order of 2 divides
n; and since n is prime, we have the order just equal to n. But also n | (q −1), the
order of the multiplicative group. q is odd so q −1 is even and we have even better
that 2n | (q − 1), or 2nk = q − 1, and the result follows.

Furthermore, Philiatrus discovered two primes n < 50 where 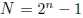is not
prime: 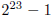is divisible by 47 and 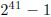is divisble by 83. Lucas proved the
following: if p = 8m + 7 is a prime, then 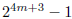is not. It can be shown that if

PERFECT NUMBERS

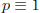or 7 modulo 8 then 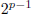− 1 is divisible by the same factors as
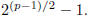
In the second case, we have (p − 1)/2 = (8m+ 7 − 1)/2 = 4m+ 3 so 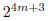− 1 by
Fermat’s little theorem is divisible by p = 8m + 7.

4. Mersenne Primes

From the previous section, we know that finding even perfect numbers reduces
simply to finding primes of the form 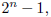where n is prime. There are 37 known
Mersenne primes, summarized in the following table [2]:

Index Even Perfect Number Number of Digits Date

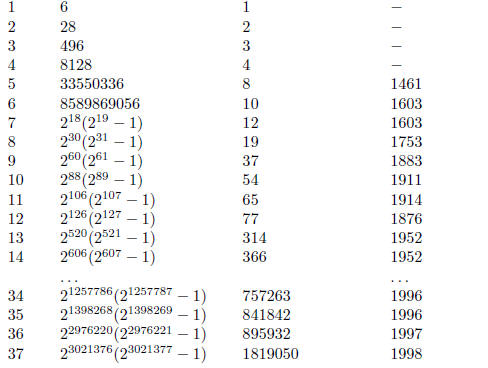
With each one of these primes, there is a story that goes with its discovery. For
instance, Peter Barlow in his Theory of Numbers published in 1811, wrote about
the eighth perfect number: “It is the greatest that will ever be discovered, for, as
they are merely curious without being useful, it is not likely that any person will
attempt to find one beyond it.”

Quite to the contrary, the latest Mersenne prime was discovered on January 27,
1998 by Roland Clarkson, a 19-year-old student at California State University–
Dominguez Hills. Roland used a 200 MHz Pentium computer part-time for 46
days to prove the number prime—he is just one of over 4000 volunteers world-wide
participating in the Great Internet Mersenne Prime Search (GIMPS). Indeed, Scott
Kurowski, the co-author of software Clarkson used to find this enormous prime, has
offered a cash prize to the discoverer of the 38th Mersenne prime. The prize pool
is $1.00 for every 1000 digits in the new prime or US $1,000.00, whichever is larger.
He says, “If you have a desktop computer, we’ve got something for it to do between
keystrokes and mouse clicks, and many more machines are needed for the next,
even larger, prime.”

JOHN VOIGHT

The Mersenne primes appear to be regularly distributed —“ballpark” estimates
(meaning within thousands of orders of magnitude) can be given [15], but ultimately
these numbers must be checked out one by one. We close with one interesting result:
Conjecture 15 (Cunningham). If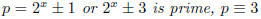 (mod 4),
and 2p + 1 is a prime, then 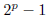is prime.