Before defining the next family of functions, the
exponential functions, we will need to
discuss exponent notation in detail. As we shall see, exponents can be used to
describe
not only powers (such as 52 and 23), but also roots (such as square roots and
cube
roots). Along the way, we’ll define higher roots and develop a few of their
properties .
More detailed work with roots will then be taken up in the next chapter.
Integer Exponents
Recall that use of a positive integer exponent is simply a shorthand for
repeated multiplication.
For example,

and

In general, bn stands for the quanitity b multiplied by
itself n times. With this definition,
the following Laws of Exponents hold.
| Laws of Exponents
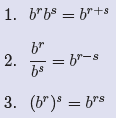 |
The Laws of Exponents are illustrated by the following
examples.
 Example 3.
Example 3.
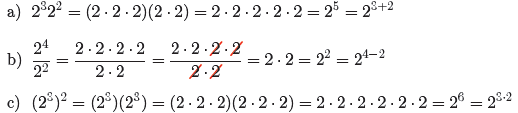
Note that the second law only makes sense for r > s, since
otherwise the exponent
r − s would be negative or 0. But actually, it turns out that we can create
definitions
for negative exponents and the 0 exponent, and consequently remove this
restriction.
Negative exponents, as well as the 0 exponent, are simply
defined in such a way that
the Laws of Exponents will work for all integer exponents.
• For the 0 exponent, the first law implies that
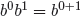 , and therefore
, and therefore
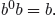 If
If
b ≠ 0, we can divide both sides by b to obtain b0 = 1 (there is one exception:
00 is
not defined).
• For negative exponents, the second law implies that
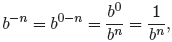
provided that b ≠ 0. For example,
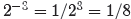 , and
, and 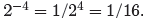
Therefore, negative exponents and the 0 exponent are defined as follows:
| Definition 4.
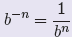 and and
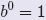
provided that b ≠ 0. |
 Example 5. Compute the exact values of
Example 5. Compute the exact values of
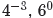 , and
, and
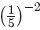 .
.
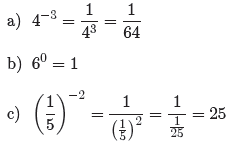
We now have bn defined for all integers n, in such a way
that the Laws of Exponents
hold. It may be surprising to learn that we can likewise define expressions
using rational
exponents, such as  , in a consistent manner. Before doing so, however, we’ll
need
, in a consistent manner. Before doing so, however, we’ll
need
to take a detour and define roots.
Roots
Square Roots : Let’s begin by defining the square root of a real number. We’ve
used the square root in many sections in this text, so it should be a familiar
concept.
Nevertheless, in this section we’ll look at square roots in more detail.
Definition 6. Given a real number a, a “square
root of a” is a number x such
that x2 = a. |
For example, 3 is a square root of 9 since 32 = 9.
Likewise, −4 is a square root of 16
since (−4)2 = 16. In a sense, taking a square root is the “opposite” of
squaring, so the
definition of square root must be intimately connected with the graph of y = x2,
the
squaring function. We investigate square roots in more detail by looking for
solutions
of the equation

There are three cases, each depending on the value and
sign of a . In each case, the
graph of the left-hand side of x2 = a is the parabola shown in Figures 1(a),
(b), and
(c).
• Case I: a < 0
The graph of the right-hand side of x2 = a is a horizontal line located a units
below
the x-axis. Hence, the graphs of y = x2 and y = a do not intersect and the
equation
x2 = a has no real solutions. This case is shown in Figure 1(a). It follows that
a
negative number has no square root.
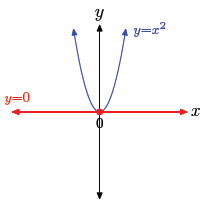
• Case II: a = 0
The graph of the right-hand side of x2 = 0 is a horizontal line that coincides
with
the x-axis. The graph of y = x2 intersects the graph of y = 0 at one point, at
the vertex of the parabola . Thus, the only solution of x 2 = 0 is x = 0, as seen
in
Figure 1(b). The solution is the square root of 0, and is denoted
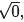 so it
follows
so it
follows
that 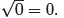
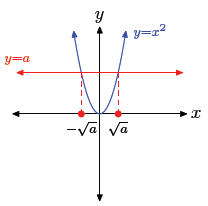
• Case III: a > 0
The graph of the right-hand side of x2 = a is a horizontal line located a units
above
the x-axis. The graphs of y = x2 and y = a have two points of intersection, and
therefore the equation x 2 = a has two real solutions, as shown in Figure 1(c).
The
solutions of x2 = a are 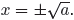 Note that we have two notations , one that calls
Note that we have two notations , one that calls
for the positive solution and a second that calls for the negative solution.
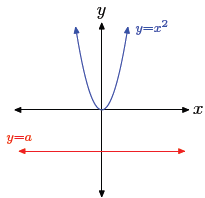
(a) No real solutions. |
(b) One real solution. |
(c) Two real solutions. |
Figure 1. The solutions of x2 = a depend upon the sign and
value of a.
Let’s look at some examples.
 Example 8. What are the solutions of x2 = −5?
Example 8. What are the solutions of x2 = −5?
The graph of the left-hand side of x2 = −5 is the parabola depicted in
Figure
1(a).
The graph of the right-hand side of x2 = −5 is a horizontal line located 5 units
below
the x-axis. Thus, the graphs do not intersect and the equation x2 = −5 has no
real
solutions.
You can also reason as follows. We’re asked to find a solution of x2 = −5, so
you
must find a number whose square equals −5. However, whenever you square a real
number, the result is always nonnegative ( zero or positive ). It is not possible
to square
a real number and get −5.
Note that this also means that it is not possible to take the square root of a
negative
number. That is, 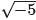 is not a real number.
is not a real number.
 Example 9. What are the solutions of x2 = 0?
Example 9. What are the solutions of x2 = 0?
There is only one solution, namely x = 0. Note that this means that
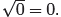
 Example 10. What are the solutions of x2 = 25?
Example 10. What are the solutions of x2 = 25?
The graph of the left-hand side of x2 = 25 is the parabola depicted in
Figure
1(c).
The graph of the right-hand side of x2 = 25 is a horizontal line located 25
units above
the x-axis. The graphs will intersect in two points, so the equation x2 = 25 has
two
real solutions.
The solutions of x2 = 25 are called square roots of 25 and are
written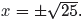
In this case, we can simplify further and write x = ±5.
It is extremely important to note the symmetry in Figure 1(c) and note that we
have two real solutions, one negative and one positive. Thus, we need two
notations,
one for the positive square root of 25 and one for the negative square root 25.
Note that (5)2 = 25, so x = 5 is the positive solution of x2 = 25. For the
positive
solution, we use the notation

This is pronounced “the positive square root of 25 is 5.”
On the other hand, note that (−5)2 = 25, so x = −5 is the negative solution of
x2 = 25. For the negative solution, we use the notation
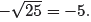
This is pronounced “the negative square root of 25 is −5.”
This discussion leads to the following detailed summary.
Summary: Square Roots
The solutions of x2 = a are called “square roots of a.”
• Case I: a < 0. The equation x2 = a has no real solutions.
• Case II: a = 0. The equation x2 = a has one real solution, namely x =
0.
Thus, 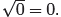
• Case III: a > 0. The equation x2 = a has two real
solutions,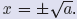 The The
notation 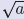 calls for the positive square root of a, that is, the positive
solution calls for the positive square root of a, that is, the positive
solution
of x2 = a. The notation 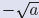 calls for the negative square root of a, that is, calls for the negative square root of a, that is,
the negative solution of x2 = a. |
Cube Roots : Let’s move on to the definition of cube roots.
Definition 11. Given a real number a, a “cube
root of a” is a number x such
that x3 = a. |
For example, 2 is a cube root of 8 since 23 = 8. Likewise,
−4 is a cube root of
−64 since (−4)3 = −64. Thus, taking the cube root is the “opposite” of cubing,
so the
definition of cube root must be closely connected to the graph of y = x3, the
cubing
function. Therefore, we look for solutions of

Because of the shape of the graph of y = x3, there is only one case to consider.
The
graph of the left-hand side of x3 = a is shown in Figure 2. The graph of the right-hand
side of x3 = a is a horizontal line, located a units above, on, or below the
x-axis,
depending on the sign and value of a. Regardless of the location of the
horizontal line
y = a, there will only be one point of intersection, as shown in Figure 2.
A detailed summary of cube roots follows.
Summary: Cube Roots
The solutions of x3 = a are called the “cube roots of a.” Whether a is
negative,
zero, or positive makes no difference . There is exactly one real
solution, namely
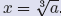 |
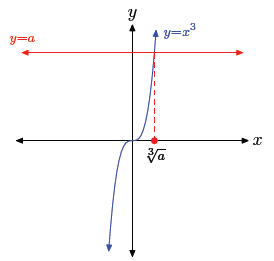
Figure 2. The graph of y = x3 intersect
the graph of y = a in exactly one
place.
Let’s look at some examples.
 Example 13. What are the solutions of x3 = 8?
Example 13. What are the solutions of x3 = 8?
The graph of the left-hand side of x3 = 8 is the cubic polynomial shown in
Figure 2.
The graph of the right-hand side of x3 = 8 is a horizontal line located 8 units
above the
x-axis. The graphs have one point of intersection, so the equation x3 = 8 has
exactly
one real solution.
The solutions of x3 = 8 are called “cube roots of 8.” As shown from the graph,
there is exactly one real solution of x3 = 8, namely
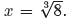 Now since (2)3 =
8, it
Now since (2)3 =
8, it
follows that x = 2 is a real solution of x3 = 8. Consequently, the cube root of
8 is 2,
and we write
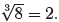
Note that in the case of cube root, there is no need for the two notations we
saw in the
square root case (one for the positive square root, one for the negative square
root).
This is because there is only one real cube root. Thus, the notation
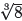 is
pronounced
is
pronounced
“the cube root of 8.”



